Math art can be very delicate and precise, so most of the time, you should use a small layer height and slow speeds. Doing this will help to preserve the model’s structure and detail.

Another way you can think about how to print math art models is to treat them like miniatures because they similarly contain a lot of detail in a small space. Our article on printing tips for miniatures is a good place to look.

For each pick below, we’ve listed some of the designer’s printing suggestions, so we recommend using their specified print settings as a starting point.

Besides serving as a neat desk toy, this first piece is a great math design that makes Sierpiński triangles into a pyramid! These triangles are fractal patterns of equilateral triangles that decrease in size and are infinitely self-similar (smaller triangles are made up of other smaller triangles, seemingly forever!).

The model forms half of a Sierpiński octahedron, according to the designer. But Sierpiński isn’t the only theorist represented in this model: The Vicsek fractal also makes an appearance in the internal layers as the model is printed.

The creator of this model states that you shouldn’t use supports, bridging, or retraction for this print. As such, it’s suggested to print it in spiral (vase) mode on your slicer and with 0% infill.

• Who made it? ricktu
• How popular/printable is it? This Sierpiński pyramid design has 304 recorded Makes, 13 Remixes, and over 136,000 downloads! So many people have printed this model, so you can too!
• Where to get it? Thingiverse

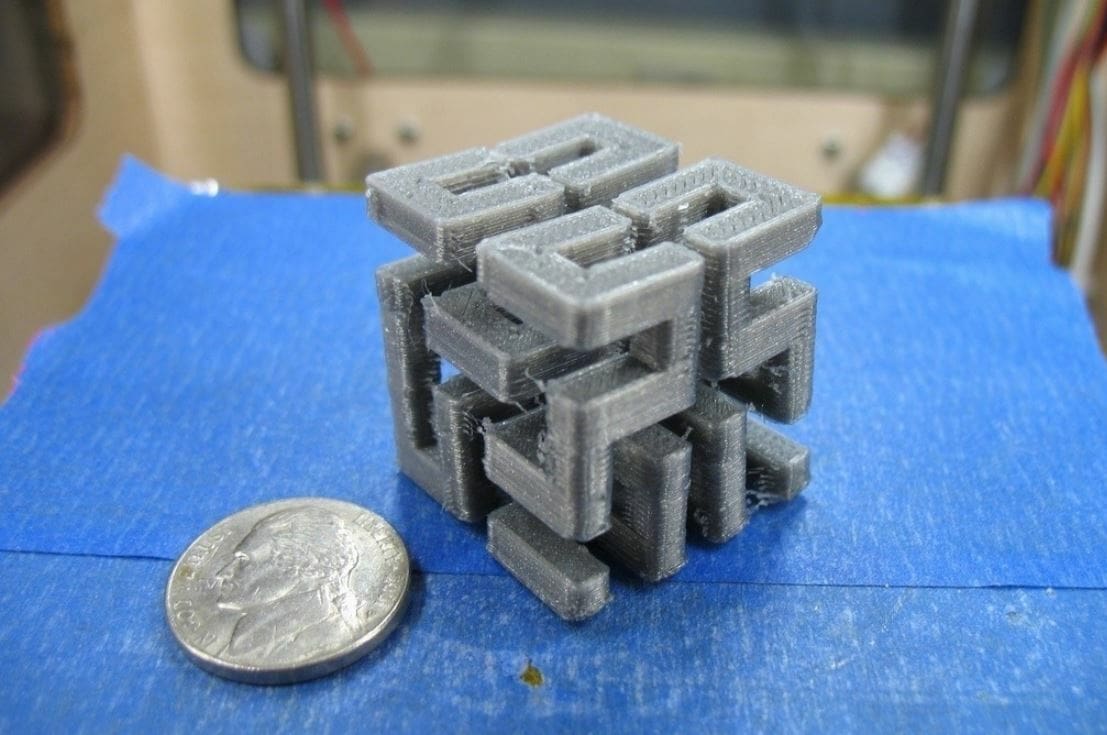
A Hilbert cube is a math art design that uses a mathematical equation to form an endless path. According to the designer, however, this design isn’t exactly a Hilbert cube because it isn’t completely continuous. The model was designed and programmed using Ruby script after the designer experienced difficulty using OpenSCAD.

The maker states that this design can only be printed in conjunction with PVA (soluble) filament for supports.

• Who made it? ErnyCrazyPrinter
• How popular/printable is it? This Hilbert cube model has over 1,100 views with 48 downloads.
• Where to get it? Cults

This next math art design is a distorted torus with a hexagonal pattern all around the piece. After using a hexagonal grid to cover a normal torus, the maker distorted it by pinching and rotating the model to yield the impressive structure and shape you see.

All of the 3D design was done in OpenSCAD and then Python was used to make the distortion. On top of the standard STL file, the designer also posted the SCAD file if you want to alter the design.

The creator of this model didn’t have a printer to make the model on their own. However, another maker posted a great print and listed that they used a 0.05-mm layer height and a 0% infill. The maker also listed that they used supports, but no raft for this print.

• Who made it? zeycus
• How popular/printable is it? This distorted torus design has 6 Makes and 1 Remix, as well as over 7,700 downloads!
• Where to get it? Thingiverse

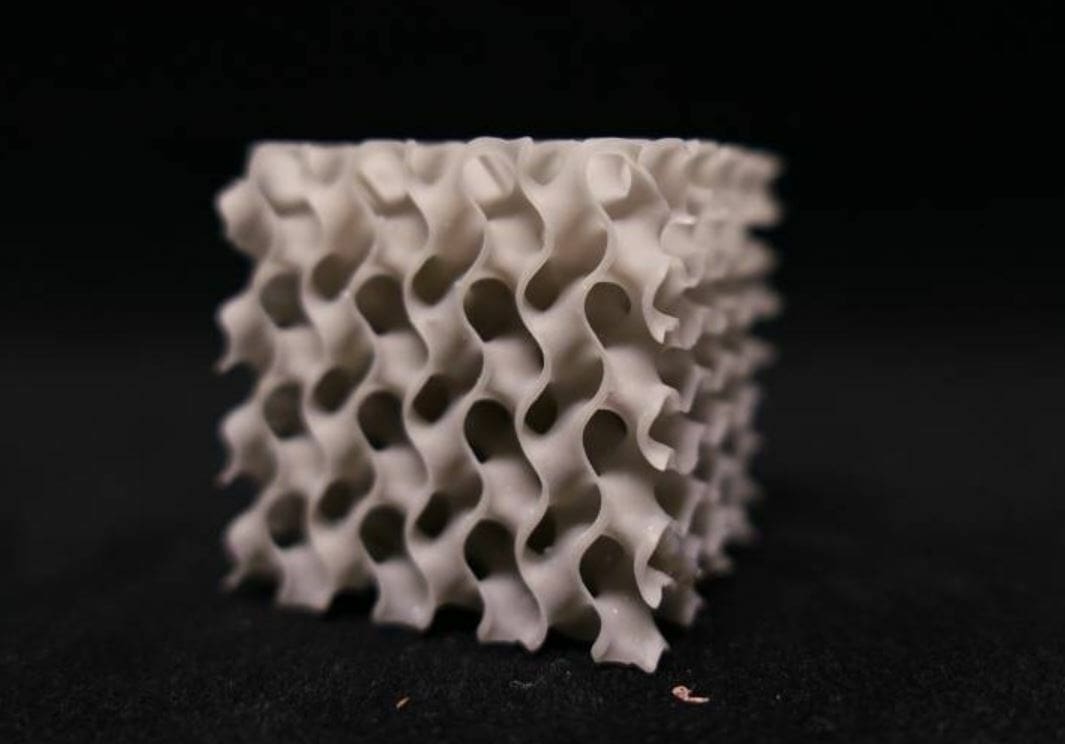
Besides being a type of infill for 3D printing, gyroid is also a structure that makes for a great piece of math art. This design is the same structure as the gyroid infill that you can use in Cura and other slicer programs. A gyroid structure is an infinitely connected triply periodic minimal surface, according to the maker.

The maker used an SLA 3D printer to make this model and states that the print has a medium-level complexity.

• Who made it? O3D Official
• How popular/printable is it? This gyroid structure model has almost 10,300 views and over 1,300 downloads.
• Where to get it? MyMiniFactory

A Klein bottle is a popular piece of fractal math art that somewhat resembles a container folding in on itself. This design is given a twist with a Voronoi pattern on its surface.

If you’re interested, the creator of this model shares how they designed the six-dimensional Klein bottle on the project page. It was a bit complicated but starts with connecting two sides of a plane to form the initial shape.

The designer suggests scaling the model up 800% in your slicer. That way, it’ll be around 12 centimeters tall. The maker also recommends printing with a large raft and a slow speed to ensure print adhesion.

• Who made it? casihonesto
• How popular/printable is it? This Klein bottle design has almost 800 views with 29 downloads.
• Where to get it? Cults

Using numerous programming languages (Grasshopper, Python, and C#) along with design software (Rhinoceros), the designer created this so-called Dugaci sculpture. The sculpture appears to be a knot and looks like it restrains itself with interlocking strands.

One maker listed that they used tree supports to successfully print this model and that they also used a raft and set the layer height at 0.1 mm. The designer also used PLA and states that the print took 17 hours.

• Who made it? bathsheba
• How popular/printable is it? There’s 1 Make as well as over 400 downloads of this cool model.
• Where to get it? Thingiverse

An oloid is a curved, geometric shape while an anti-oloid is a modified version of an oloid. Besides looking cool, this anti-oloid rolls on its own with a perfect center.

The designer was inspired to make this math art model by a Maker’s Muse video about anti-oloids. They then used Fusion 360 to model this piece.

It’s suggested to print this anti-oloid with a 5% infill, a 0.48-mm layer height, and scaled to 200%.. The designer also recommends not using supports but that you’ll want to activate a brim if you don’t alter the scale of the model. They successfully printed it in PLA on a CR-10S.

Check it out in action!

• Who made it? SpikeUK
• How popular/printable is it? This anti-oloid design has 7 recorded makes and over 3,700 downloads!
• Where to get it? Thingiverse

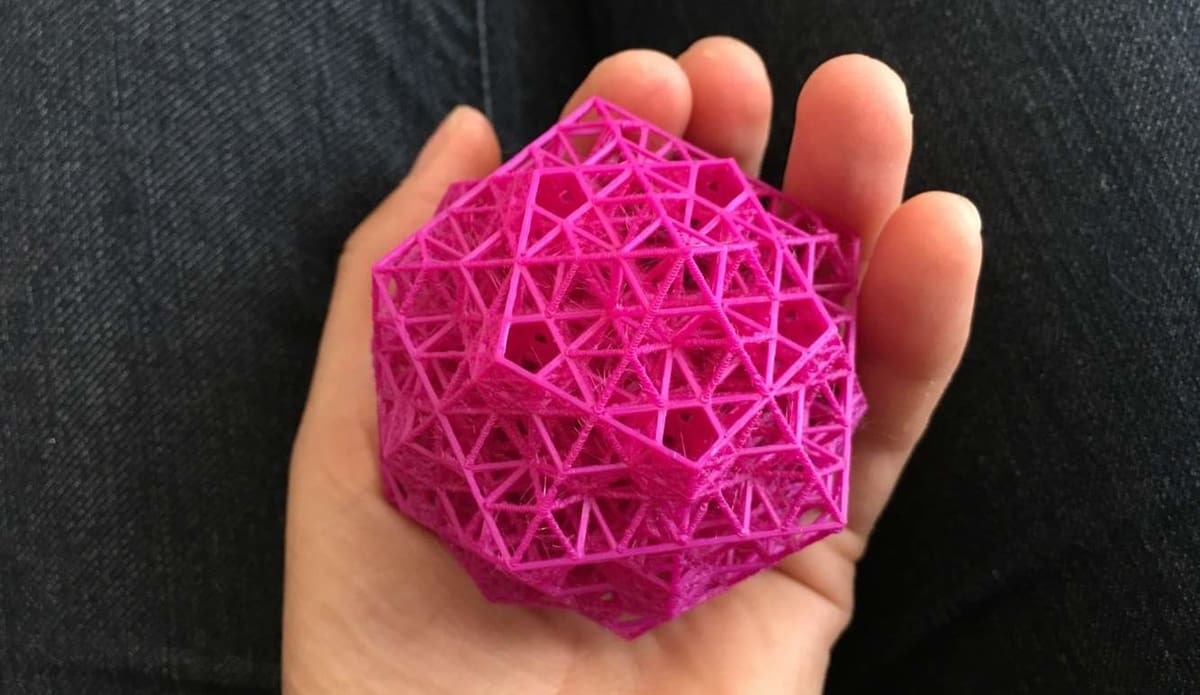
This quasi-crystalline spin network (QSN) is definitely one of the more complex math art selections on this list. Given its intricacies, the QSN can be classified as a 3D printer torture test in addition to being math art.

The structure is based on a cuboctahedron with many triangular and square faces. According to the designer, these faces don’t ever touch each other. The cuboctahedron shape was transformed and altered, which you can read about on the project page, or learn more about it in the explanation video.

The designer suggests printing with a 0.175- to 0.2-mm layer height for larger models and using a 0% infill.

• Who made it? RWGresesrch
• How popular/printable is it? This QSN model has 1 community print, over 10,800 views, and almost 700 downloads!
• Where to get it? MyMiniFactory

A golden triangle, also known as a logarithmic spiral, is another popular type of math art that contains some unique geometry. This specific piece incorporates the golden ratio, mathematically referred to as ϕ, and an isosceles triangle. The maker has posted numerous sizes of the triangle, which means you won’t need a large printer to make one.

The designer recommends printing with a 5%-20% infill, and neither a raft nor supports are needed.

• Who made it? lgbu
• How popular/printable is it? This golden spiral design has 1 recorded make as well as over 500 downloads.
• Where to see it? Thingiverse

A Menger sponge is yet another well-known form of math art. A Menger sponge is also referred to as Sierpiński’s cube and is essentially a cubic version of the pyramid we saw above, following the same pattern of infinitely self-similar shapes, just with squares instead of triangles!

This fractal model was designed using OpenSCAD and Blender. One maker provided some printing advice based on their experience, listing that they used high-resolution settings, no raft, and no supports.

• Who made it? taroh
• How popular/printable is it? This Menger sponge model has 2 recorded Makes, 1 Remix, and over 2,200 downloads.
• Where to see it? Thingiverse

Pi is perhaps the most famous symbol of math, symbolized as π.  and defines the ratio of a circle’s circumference to the circle’s diameter. The constant is an infinite number that goes like 3.14159265359… just kidding, we won’t go on forever! This final piece of math art is a Pi pen holder that uses Pi’s first many decimal places as the sides of the cup.

Many Thingiverse users have posted great prints and helpfully provided their settings. One maker suggests printing with a standard 0.2-mm layer height and a 100% infill. The designer printed this themself using PLA, though resin or glow-in-the-dark filament is also an option!

• Who made it? roman_hegglin
• How popular/printable is it? This design has an amazing 284 recorded Makes, 12 Remixes, and over 171,000 downloads! So many others have successfully printed this model, so you can make it too!
• Where to see it? Thingiverse